Circular Motion Calculator

Our circular motion calculator is here to help you calculate essential parameters of an object in a uniform circular motion, such as frequency, speed, angular velocity, and centripetal acceleration. In this article, let's learn more about objects moving in a circle at a constant speed. Specifically, let's look at:

• What is uniform circular motion?
• Uniform circular motion formulae.
• An example of circular motion calculation.

Picture an object such as a small stone or your keys tied to a string. When you whirl it above your head (somewhere safe, of course), the object moves in a circular path. If the object's speed (magnitude of its velocity) stays constant throughout, then we term it as uniform circular motion.

The changing velocity results from the centripetal force acting on the body, which keeps the motion in a circular path. The acceleration acting on the body is called centripetal acceleration.

Consider an object traveling around the rim of a circle with a radius $r$ and a center $O$ (see figure 2). Let's figure out the various parameters of this uniform circular motion.

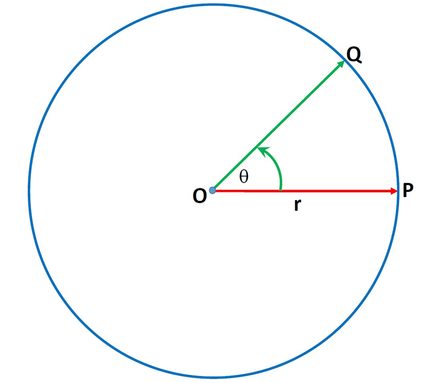
1. Angular displacement is a measure of the angle $\theta$ made by the radius $r$ at the center at any point from its initial position. It is measured in radians $(\text{rad})$.

2. Time period of circular motion $T$ is the time it takes to complete one revolution. It is measured in seconds $(\text{s})$.

3. Frequency $f$ is the number of revolutions completed in one second. It is the reciprocal of the time period of circular motion$T$. It is measured in Hertz $(\text{Hz})$.

6. Speed $v$ is the magnitude of velocity in the circular motion, which remains constant in a uniform circular motion. The SI unit of speed is meters per second $\text{m/s}$. For one revolution along a circular path of radius $r$, velocity is given by:

5. Angular velocity $\omega$ is the time rate of change of angular displacement. Measured in radians per second $(\text{rad/s})$, the angular velocity in circular motion is given by:

If the angular displacement is $2\pi$, i.e., one revolution, then the time taken is equal to the time period $T$, which leads to:

6. Angular acceleration $\alpha$ is the time rate of change of angular velocity. Measured in radians per second squared $(\text{rad/s}^2)$, the angular acceleration in circular motion is given by:

7. Centripetal acceleration $\alpha_c$ is the acceleration that keeps the object moving in a circular path. It is given by:

We can better understand how to calculate circular motion with the help of an example. Imagine you're riding a carousel at a carnival. Let's say your seat is 6 meters from its center. At its maximum speed, you complete one revolution in 4 seconds. Calculate the centripetal acceleration you experience in this ride.

What we know:

• Radius of the circular motion $r = 6 \text{ m}$.
• Period of the circular motion $T = 4 \text{ m}$.

The frequency of the circular motion would be:

The speed (velocity) of the circular motion is given by:

While its angular velocity is:

We can now calculate the centripetal acceleration using the speed and radius:

Which is about 1.5 times the gravitational acceleration.

This uniform circular motion calculator operates in two modes:

1. Calculating time period, frequency, and angular velocity:
   • Enter any one of these parameters with appropriate units.
   • The calculator can determine the remaining two parameters automatically.
2. Determining the radius, speed, and acceleration:
   • Provide the circular motion's time period, frequency, or angular velocity.
   • Enter the value of the radius, speed, or centripetal acceleration.
   • The circular motion calculator will determine the rest of the parameters with these two inputs.